Study Exponential and Inverse Functions in Detail

An exponential and Inverse function are mathematical functions, which are used in many real-world situations. The Exponential Function has usage in finding exponential decay or exponential growth or to compute investments, model populations and so on. Whereas, inverse functions are used for input and output in computers, converting temperature from Fahrenheit to Celsius and so on.

Let’s learn more about various concepts of Exponential and Inverse Functions from types and properties to the steps to find them with proper examples for better understanding.

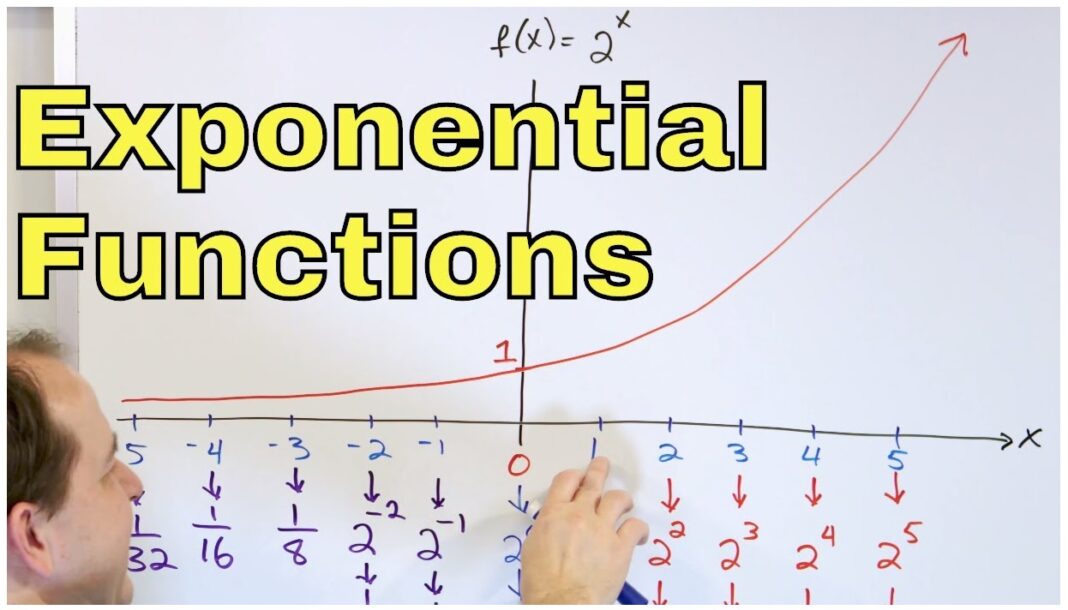
What is Exponential Function?

An exponential function is a function of form f (x) = a^x, where “x” is a variable and “a” is a constant that is considered the function’s base and should be greater than 0.

Here are some examples of exponential functions.

f(x) = 3^x

f(x) = (1/2)^x

f(x) = 3e^2x

f(x) = 4 (3)^-0.5x

Exponential Function Formula

The formula f(x) = a^x defines an exponential function, where x is the input variable as an exponent. 

The exponential function is a crucial mathematical function of the form

f(x) = a^x

Where,

x is any real number

a>0 and not equal to 1.

Extending the possibilities of many exponential functions, an exponential function may include a constant as a multiple of the variable in its power. In other words, an exponential function can also take the form f(x) = e^kx. It can also have the form f(x) = p.e^kx, where p is a constant.

When the value of a quantity increases in exponential growth and drops in an exponential decay, the exponential function appears.

Exponential Function Rules

The rules of an exponential function are identical to the rules of exponents. Here are several exponent rules.

• a⁰ = 1
• a^m × aⁿ = a^(m+n)
• a^m/aⁿ = a^(m-n)
• (a^m)ⁿ = a^mn
• (ab)^m = a^mb^m
• (a/b)^m = a^m/b^m
• a^-m = 1/a^m

Inverse Function

A function that can be reversed into another is known as an inverse function, sometimes known as an anti function. Simply, if a function “f” transforms x into y, its inverse transforms y into x. If the function is denoted by ‘f’ or ‘F,’ the inverse function is denoted by f^-1 or F^-1.

F and g can only be inverse functions of each other, if f(x) = y if and only if g(y) = x.

For example: f(x) = 2x + 5 = y

The inverse of f is hence g(y) = (y-5)/2 = x. (x).

Types of Inverse Function

Let’s discuss inverses of some of the most common functions.

Inverse Trigonometric Functions

The inverse trigonometric functions are also known as arc functions as they produce the length of the arc, which is required to obtain that particular value. Arcsine (sin^-1), arccosine (cos^-1), arctangent (tan^-1), arcsecant (sec^-1), arccosecant (cosec^-1), and arccotangent (cot^-1) are the six inverse trigonometric functions.

Inverse Rational Function

A rational function is one with the form f(x) = P(x)/Q(x), where Q(x) = 0.

Inverse Hyperbolic Functions

Inverse hyperbolic functions, like inverse trigonometric functions, are the inverses of hyperbolic functions. sinh^-1, cosh^-1, tanh^-1, coth^-1, Cosech^-1 and sech^-1 are some common inverse hyperbolic functions.

Inverse Logarithmic Functions and Inverse Exponential Function

The exponential functions are the inverse of the natural log functions.

Steps To Find An Inverse Function

The steps below will help you in quickly determine the inverse of a function. Here, we take the function f(x) = ax+b and attempt to discover its inverse.

• Replace f(x) = y with the given function f(x) = ax + b to get y = ax+ b.
• Substitute x with y and y with x in the function y = axe + b to get x = ay + b.
• Now, solve for y the expression x = ay + b. Hence, we get y = (x – b/a).
• Finally, we can substitute y = f^-1(x) with f^-1(x) = (x – b)/a.